Blog 2 (Gears Documentation)

WHAT'S GOOD EVERYBODY😸😸! Welcome to my second blog documentation about GEARS. Without further ado, lets get straight into it. Sit back, relax and enjoy!

In this blog, I will document the following:

1. The definition of gear module, pitch circular diameter and the relationship between gear module, pitch circular diameter and number of teeth.
2. The relationship between gear ratio (speed ratio) and output speed, between gear ratio and torque for a pair of gears.
3. How can I design a better hand-squeezed fan, including the sketches.
4. How my practical team arranged the gears provided in the practical to raise the water bottle, consisting of:

• Calculation of the gear ratio (speed ratio)
• The photo of the actual gear layout.
• Calculation of the number of revolutions required to rotate the crank handle.
• The video of the turning of the gears to lift the water bottle.

      5. My Learning reflection on the gear activities. 

Before I start, let me give y'all a quick introduction about GEARS. The picture on the right depicts a gear train and the different type of gears. A COMPOUND is a gear that consists of a number of gears fixed together. Therefore, they rotate at the same speed despite the different amount of teeth each of the gear has. An IDLER is a gear that is placed in between 2 other gears to transmit motion from one to the other. It does not affect the speed of the output but it does affect the direction it turns. So now you all know a little more about what GEARS are, lets continue.

1. These are the definition of gear module, pitch circular diameter and the relationship between the gear module, pitch circular diameter and number of teeth:

Different kinds of Gear Module

Gear Module - Gear module refers to the size of the gear teeth. It is the ratio of the reference diameter of the gear divided by the number of teeth it has. The unit for gear module is millimeter (mm). Gears can only be connected together if they have the same gear module.

Pitch Circular Diameter (PCD) - It refers to the imaginary circle that passes through the contact point between two meshing gears. It represents the diameters of two friction rollers in contact and moves at the same linear velocity.

Relationship between the gear module, PCD and number of teeth - 

Gear Module (m)  = PCD ÷ Number of Teeth (z)

2. Below is the relationship between gear ratio (speed ratio) and output speed for a pair of gears.

Relationship Between Gear Ratio & Speed Ratio

Gear Ratio = Output ÷ Input , Speed Ratio = Input ÷ Output , Therefore, Gear Ratio = 1 ÷ Speed Ratio

Relationship between gear ratio (speed ratio) and output speed for a pair of gears
Speed of Output = Speed of Input ÷ Gear Ratio

If the speed ratio is larger than 1.0, then the gear pair is operating as speed increaser. If the speed ratio is between 0.0 and 1.0, then the gear pair is operating as a speed reducer.

   Below is the relationship between gear ratio and torque for a pair of gears.

Torque is the measure of twisting force, calculated as the product of circumferential force multiplied by the radius of the gear. This means that bigger gears will have more torque than smaller gears because of the larger radii of the gears.

Gear Ratio = Output Torque ÷ Input Torque. Hence, a larger gear ratio will result in a larger output torque.

3. Below are the proposed design to make the hand-squeezed fan better:

Initial Design of the hand-squeezed fan

Proposed Design

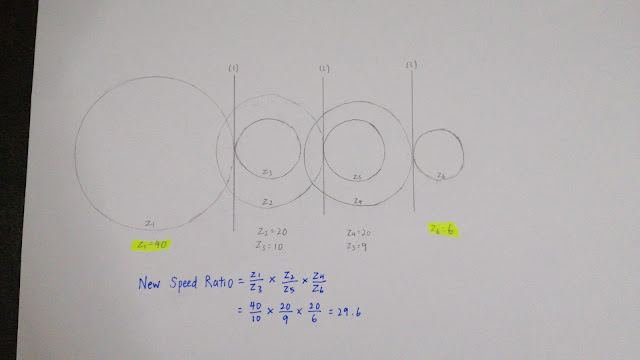
A proposed design to make the hand-squeezed fan better is to increase the size of the input gear. As we know speed ratio is input/output, increasing the size of the input gear will increase the speed of the output gear, causing the fan to rotate more rounds. We can also decrease the size of the output gear to increase the speed ratio. 

The old speed ratio is (20÷10) x (20÷9) x (20÷9) = 9.88

By changing the size of the input gear to be bigger and output gear to be smaller, the speed ratio is tripled. This means that every time the handle is cranked, the turbine will be able to rotate 29.6 times!

4. Below are the description on how my practical team arranged the gears provided in the practical to raise the water bottle.

a. Calculation of the gear ratio (speed ratio).

As our task is to lift the water bottle up by 20cm, our group's aim is to achieve a higher output torque. Since gear ratio = output torque/ input torque, we would need a high gear ratio. Hence, my group came up with the design show on the right. 

With the calculated gear ratio, speed ratio = 1/gear ratio = 1/26.7 = 0.0375

b. The photo of the actual gear layout.

c. Calculation of the number of revolutions required to rotate the crank handle.

d. The video of the turning of the gears to lift the water bottle.

5. Below is my Learning Reflection on the gears activities.

Before the practical day, we were told to watch 4 videos on Brightspace so that we would be more equipped for the activities next day. Before watching the videos, the only thing I know about gears are that they are used in bicycles. After studying through the 4 videos, I got to know more about gears and the principles and concept behind them. I learnt terms such as gear ratio, speed ratio, gear module, torque etc... which came in very handy for the practical day as we had to use those terms to complete our tasks. 

During the practical day, I was very excited to meet my group members as these people will be my groupmates for the next few practical. After a few minutes of recap on what gears are, we went off to start our activities. Our group decided to split our work such that 1 person will do the group worksheet, while the other 4 will start on brainstorming on how to conquer the activities. Using the knowledge we had gain from the videos, we knew that we need a higher output torque to lift the water bottle up by 20cm, our group decided to arrange the gears such that we will get the maximum gear ratio as a higher gear ratio will produce a higher output torque. My groupmate, Aminur came out with a brilliant idea to use a scotch tape to label the different types of gears according to their number of teeth so that it will be easier and convenient for us to arrange our compound gear train. After a few tries, we managed to achieve a whopping 26.7 gear ratio which is the highest among the 4 groups. We were really proud of what we had accomplished.

For the second activity, we were required to assemble the hand-powered fan. During this activity, I followed the picture given and started building it. However, after finish building it, the hand-powered fan did not work as the handle cannot be cranked. As we were given 2 sets of the hand-powered fan, I quickly started assembling the 2nd set and tried to crank the handle again. It did not work. I felt frustrated as I spent quite long finding the mistake on what I did wrong but I could not. After consulting with the technical executive, Ms Serene, we were told to lend a set from the other team and when we use their set, it worked! After that, I calculated the gear ratio (speed ratio) and compared if the number of rotation of the turbine is similar with my calculations. It is quite similar as we managed to achieve 10 rotations per handle cranked and our calculation of the speed ratio is 9.88. From this activity learnt that our sets did not work may due to the parts as they are 3D printed, it will not be perfect. In addition, I learnt that we should during times of failure and we should keep trying until we succeed. 

In conclusion, this practical was a blast and I hope that I will be able to work with gears again as it made us use divergent thinking to solve the challenges we had. After this practical, my knowledge about gears has expanded and in the future, if I am working with any gears, I would at least have some knowledge about how it works and the principle and concepts behind it.